add
add longest videos
Loading...
Loading...
Loading...
Branches of Mathematics
Pure mathematics
Applied mathematics
- Dynamical systems and differential equations
- Mathematical physics
- Computing
- Information theory and signal processing
- Probability and statistics
- Game theory
- Operations research
Related Topics
- Electromagnetic Spectrum
- Linear Equations
- Applied Physics
- Nano Technology
- Engineering Sciences
- Mathematical Medicine
- Fluid dynamics
- Computational Mathematics
- Gamma Ray Optics
- Onfrared Optics
- Relative Velocity
- Iciam
- Intelligent Imaging Neuroscience
- Biomedical Engineering
- Neuroscience Research
- Quantum Cosmology
- Brain Research
Open Collage in a new Window
Email the Collage
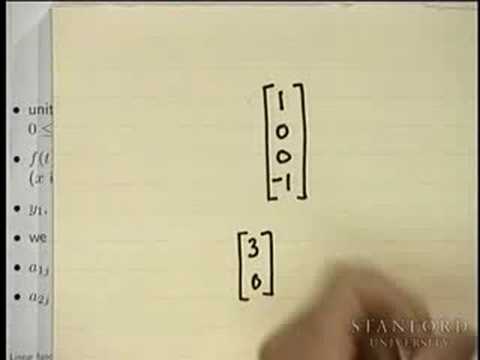
A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders. Differential equations play a prominent role in engineering, physics, economics, and other disciplines.
Differential equations arise in many areas of science and technology, specifically whenever a deterministic relation involving some continuously varying quantities (modeled by functions) and their rates of change in space and/or time (expressed as derivatives) is known or postulated. This is illustrated in classical mechanics, where the motion of a body is described by its position and velocity as the time value varies. Newton's laws allow one (given the position, velocity, acceleration and various forces acting on the body) to express these variables dynamically as a differential equation for the unknown position of the body as a function of time. In some cases, this differential equation (called an equation of motion) may be solved explicitly.
This page contains text from Wikipedia, the Free Encyclopedia -
http://en.wikipedia.org/wiki/Differential_equation
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a lake.
At any given time a dynamical system has a state given by a set of real numbers (a vector) that can be represented by a point in an appropriate state space (a geometrical manifold). Small changes in the state of the system create small changes in the numbers. The evolution rule of the dynamical system is a fixed rule that describes what future states follow from the current state. The rule is deterministic; in other words, for a given time interval only one future state follows from the current state.
The concept of a dynamical system has its origins in Newtonian mechanics. There, as in other natural sciences and engineering disciplines, the evolution rule of dynamical systems is given implicitly by a relation that gives the state of the system only a short time into the future. (The relation is either a differential equation, difference equation or other time scale.) To determine the state for all future times requires iterating the relation many times—each advancing time a small step. The iteration procedure is referred to as solving the system or integrating the system. Once the system can be solved, given an initial point it is possible to determine all its future positions, a collection of points known as a trajectory or orbit.
This page contains text from Wikipedia, the Free Encyclopedia -
http://en.wikipedia.org/wiki/Dynamical_system
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
- Loading...
Loading suggestions ...
-
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Lec 27 | MIT 18.03 Differential Equations, Spring 2006 -
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Lec 31 | MIT 18.03 Differential Equations, Spring 2006Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the Non-linear Pendulum. View the complete course: http://ocw.mit.edu/... -
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
System Dynamics and Control: Module 3 - Mathematical Modeling Part ISystem Dynamics and Control: Module 3 - Mathematical Modeling Part I
Discussion of differential equations as a representation of dynamic systems. Introduction to the Laplace Transform as a tool for solving differential equations. -
Multiagent Dynamical Systems
Multiagent Dynamical SystemsMultiagent Dynamical Systems
I will show how to model multiagent systems using dynamical systems theory by deriving a class of macroscopic differential equations that describe mutual ada... -
Fixed points and stability of a nonlinear system
Fixed points and stability of a nonlinear systemFixed points and stability of a nonlinear system
Find the fixed points and determines the linear stability of a system of two first-order nonlinear differential equations. Lecture notes at http://www.math.u... -
Dynamical Systems
Dynamical SystemsDynamical Systems
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - chaos, fractals, networks, power laws. What do these terms mean in a r... -
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and SystemsLecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim View the complete course: http://ocw.mit.edu/RES-6.007S11 License: Cre... -
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation ( Dynamic Systems ) | Mechanical EngineeringState Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation Dynamic Systems. -
Logical Analysis of Hybrid Systems
Logical Analysis of Hybrid SystemsLogical Analysis of Hybrid Systems
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Department, Carnegie Mellon University Hybrid systems model cyber-physical ... -
Lecture 2 | Introduction to Linear Dynamical Systems
Lecture 2 | Introduction to Linear Dynamical SystemsLecture 2 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on linear functions for the course, Introduction to Linear ... -
Lecture 3 | Introduction to Linear Dynamical Systems
Lecture 3 | Introduction to Linear Dynamical SystemsLecture 3 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives a review of linear algebra for the course, Introduction to Lin... -
Lecture 6 | Introduction to Linear Dynamical Systems
Lecture 6 | Introduction to Linear Dynamical SystemsLecture 6 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on the applications of least squares for the course, Introd... -
Lecture 9 | Introduction to Linear Dynamical Systems
Lecture 9 | Introduction to Linear Dynamical SystemsLecture 9 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems for the course, Intr... -
Lecture 10 | Introduction to Linear Dynamical Systems
Lecture 10 | Introduction to Linear Dynamical SystemsLecture 10 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems and how they relate ...
- Biology
- Black–Scholes
- Cartesian product
- Chaos theory
- Chemistry
- Classical mechanics
- Complex analysis
- Dependent variable
- Derivative
- Difference equation
- Difference equations
- Differentiable
- Diffusion
- Dynamical systems
- Dynamics (mechanics)
- Economics
- Electromagnetism
- Engineering
- Equation
- Equations of motion
- Fluid dynamics
- Fractional calculus
- General relativity
- Geodesic
- Hamilton's equations
- Harmonic function
- Harmonic oscillator
- Heat
- Heat equation
- Hodgkin–Huxley model
- Implicit function
- Integral equations
- Integral transform
- Integrating factor
- Joseph Fourier
- Lamar University
- Laplace equation
- Laplace's equation
- Linear combination
- Linearization
- Lorenz attractor
- Map (mathematics)
- Mathematics
- Matrix (mathematics)
- Maxima (software)
- Maxwell's equations
- Meteorology
- MIT
- Molecular dynamics
- Newton's Second Law
- Nuclear physics
- Numerical methods
- Partial derivatives
- Pendulum
- Physics
- Poisson's equation
- Pure mathematics
- Quantum mechanics
- Radioactive decay
- Real numbers
- Replicator dynamics
- Schrödinger equation
- Set (mathematics)
- Sethi model
- Stability theory
- Steady state
- Stochastic process
- Symmetries
- Thermodynamics
- Time scale calculus
- UIUC
- Vector space
- Verhulst equation
- Von Bertalanffy
- Wave equation
- Weak derivative
- Weak solution
- Wiener process
-
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Lec 27 | MIT 18.03 Differential Equations, Spring 2006Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Sketching Solutions of 2x2 Homogeneous Linear System with Constant Coefficients View the complete course: http://ocw.mit.edu/18-03S06 License: Creative Commo... -
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Lec 31 | MIT 18.03 Differential Equations, Spring 2006Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the Non-linear Pendulum. View the complete course: http://ocw.mit.edu/... -
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
System Dynamics and Control: Module 3 - Mathematical Modeling Part ISystem Dynamics and Control: Module 3 - Mathematical Modeling Part I
Discussion of differential equations as a representation of dynamic systems. Introduction to the Laplace Transform as a tool for solving differential equations. -
Multiagent Dynamical Systems
Multiagent Dynamical SystemsMultiagent Dynamical Systems
I will show how to model multiagent systems using dynamical systems theory by deriving a class of macroscopic differential equations that describe mutual ada... -
Fixed points and stability of a nonlinear system
Fixed points and stability of a nonlinear systemFixed points and stability of a nonlinear system
Find the fixed points and determines the linear stability of a system of two first-order nonlinear differential equations. Lecture notes at http://www.math.u... -
Dynamical Systems
Dynamical SystemsDynamical Systems
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - chaos, fractals, networks, power laws. What do these terms mean in a r... -
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and SystemsLecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim View the complete course: http://ocw.mit.edu/RES-6.007S11 License: Cre... -
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation ( Dynamic Systems ) | Mechanical EngineeringState Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation Dynamic Systems. -
Logical Analysis of Hybrid Systems
Logical Analysis of Hybrid SystemsLogical Analysis of Hybrid Systems
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Department, Carnegie Mellon University Hybrid systems model cyber-physical ... -
Lecture 2 | Introduction to Linear Dynamical Systems
Lecture 2 | Introduction to Linear Dynamical SystemsLecture 2 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on linear functions for the course, Introduction to Linear ... -
Lecture 3 | Introduction to Linear Dynamical Systems
Lecture 3 | Introduction to Linear Dynamical SystemsLecture 3 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives a review of linear algebra for the course, Introduction to Lin... -
Lecture 6 | Introduction to Linear Dynamical Systems
Lecture 6 | Introduction to Linear Dynamical SystemsLecture 6 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on the applications of least squares for the course, Introd... -
Lecture 9 | Introduction to Linear Dynamical Systems
Lecture 9 | Introduction to Linear Dynamical SystemsLecture 9 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems for the course, Intr... -
Lecture 10 | Introduction to Linear Dynamical Systems
Lecture 10 | Introduction to Linear Dynamical SystemsLecture 10 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems and how they relate ... -
Lecture 11 | Introduction to Linear Dynamical Systems
Lecture 11 | Introduction to Linear Dynamical SystemsLecture 11 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on how to find solutions via LaPlace transform and the use ... -
Lecture 12 | Introduction to Linear Dynamical Systems
Lecture 12 | Introduction to Linear Dynamical SystemsLecture 12 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on matrix exponentials, eigenvectors, and diagonalization a... -
Lecture 13 | Introduction to Linear Dynamical Systems
Lecture 13 | Introduction to Linear Dynamical SystemsLecture 13 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on generalized eigenvectors, diagonalization, and Jordan ca... -
Lecture 15 | Introduction to Linear Dynamical Systems
Lecture 15 | Introduction to Linear Dynamical SystemsLecture 15 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on inputs and outputs of linear dynamical systems, as well ... -
Homogeneous Systems of Linear Equations - Intro to Eigenvalue/Eigenvector Method
Homogeneous Systems of Linear Equations - Intro to Eigenvalue/Eigenvector MethodHomogeneous Systems of Linear Equations - Intro to Eigenvalue/Eigenvector Method
Gives an overview of the notation and terminology used when working with linear systems of differential equations. Outlines the method to be used for finding eigenvalues and eigenvectors, which provide solutions for a system of equations. -
Differential Equations - Solve Linear System using Laplace transforms
Differential Equations - Solve Linear System using Laplace transformsDifferential Equations - Solve Linear System using Laplace transforms
Solve the system: dx/dt + 3x + dy/dt = 1, dx/dt - x + dy/dt - y = e^t, x(0)=0, y(0)=0. -
Linearizing non-linear dynamic equations
Linearizing non-linear dynamic equationsLinearizing non-linear dynamic equations
In this lecture, we go through the steps of linearizing non-linear differential equations about a given operating/equilibrium point. We will use the multi-va... -
Partial differential equation
Partial differential equationPartial differential equation
In mathematics, a partial differential equation (PDE) is a differential equation that contains unknown multivariable functions and their partial derivatives.... -
Differential Equations, The Exponential Map Perspective - Lecture 7
Differential Equations, The Exponential Map Perspective - Lecture 7Differential Equations, The Exponential Map Perspective - Lecture 7
The seventh in a series of lectures which will examine differential equations from the perspective of the exponential map. The seventh lecture continues with...
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
- Duration: 50:27
- Updated: 03 Sep 2014
Sketching Solutions of 2x2 Homogeneous Linear System with Constant Coefficients View the complete course: http://ocw.mit.edu/18-03S06 License: Creative Commo...
http://wn.com/Lec_27_|_MIT_18.03_Differential_Equations,_Spring_2006
Sketching Solutions of 2x2 Homogeneous Linear System with Constant Coefficients View the complete course: http://ocw.mit.edu/18-03S06 License: Creative Commo...
- published: 17 Jan 2008
- views: 52637
- author: MIT OpenCourseWare
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
- Duration: 47:11
- Updated: 26 Jul 2014
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the Non-linear Pendulum. View the complete course: http://ocw.mit.edu/...
http://wn.com/Lec_31_|_MIT_18.03_Differential_Equations,_Spring_2006
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the Non-linear Pendulum. View the complete course: http://ocw.mit.edu/...
- published: 17 Jan 2008
- views: 33293
- author: MIT OpenCourseWare
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
- Duration: 65:34
- Updated: 25 Aug 2014
Discussion of differential equations as a representation of dynamic systems. Introduction to the Laplace Transform as a tool for solving differential equations.
http://wn.com/System_Dynamics_and_Control_Module_3_-_Mathematical_Modeling_Part_I
Discussion of differential equations as a representation of dynamic systems. Introduction to the Laplace Transform as a tool for solving differential equations.
- published: 18 Aug 2013
- views: 4699
- author: Rick Hill
Multiagent Dynamical Systems
- Duration: 56:46
- Updated: 27 Aug 2014
I will show how to model multiagent systems using dynamical systems theory by deriving a class of macroscopic differential equations that describe mutual ada...
http://wn.com/Multiagent_Dynamical_Systems
I will show how to model multiagent systems using dynamical systems theory by deriving a class of macroscopic differential equations that describe mutual ada...
- published: 04 May 2012
- views: 1356
- author: Bill Broadley
Fixed points and stability of a nonlinear system
- Duration: 18:01
- Updated: 21 Jun 2014
Find the fixed points and determines the linear stability of a system of two first-order nonlinear differential equations. Lecture notes at http://www.math.u...
http://wn.com/Fixed_points_and_stability_of_a_nonlinear_system
Find the fixed points and determines the linear stability of a system of two first-order nonlinear differential equations. Lecture notes at http://www.math.u...
- published: 11 Nov 2013
- views: 2789
- author: Prof. Jeffrey Chasnov
Dynamical Systems
- Duration: 101:13
- Updated: 26 Feb 2014
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - chaos, fractals, networks, power laws. What do these terms mean in a r...
http://wn.com/Dynamical_Systems
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - chaos, fractals, networks, power laws. What do these terms mean in a r...
- published: 27 Oct 2012
- views: 3436
- author: Stanford Complexity Group
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
- Duration: 47:15
- Updated: 04 Sep 2014
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim View the complete course: http://ocw.mit.edu/RES-6.007S11 License: Cre...
http://wn.com/Lecture_6,_Systems_Represented_by_Differential_Equations_|_MIT_RES.6.007_Signals_and_Systems
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim View the complete course: http://ocw.mit.edu/RES-6.007S11 License: Cre...
- published: 02 Sep 2011
- views: 16138
- author: MIT OpenCourseWare
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
- Duration: 9:01
- Updated: 04 Sep 2014
State Space Representation Dynamic Systems.
http://wn.com/State_Space_Representation_(_Dynamic_Systems_)_|_Mechanical_Engineering
Logical Analysis of Hybrid Systems
- Duration: 53:31
- Updated: 01 Apr 2014
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Department, Carnegie Mellon University Hybrid systems model cyber-physical ...
http://wn.com/Logical_Analysis_of_Hybrid_Systems
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Department, Carnegie Mellon University Hybrid systems model cyber-physical ...
- published: 17 Mar 2011
- views: 972
- author: cmurobotics
Lecture 2 | Introduction to Linear Dynamical Systems
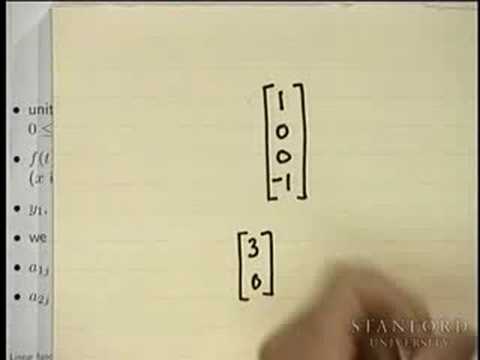
- Duration: 65:52
- Updated: 04 Sep 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on linear functions for the course, Introduction to Linear ...
http://wn.com/Lecture_2_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on linear functions for the course, Introduction to Linear ...
- published: 09 Jul 2008
- views: 45047
- author: Stanford
Lecture 3 | Introduction to Linear Dynamical Systems
- Duration: 79:11
- Updated: 18 Aug 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives a review of linear algebra for the course, Introduction to Lin...
http://wn.com/Lecture_3_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives a review of linear algebra for the course, Introduction to Lin...
- published: 09 Jul 2008
- views: 28340
- author: Stanford
Lecture 6 | Introduction to Linear Dynamical Systems
- Duration: 76:19
- Updated: 02 Jul 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on the applications of least squares for the course, Introd...
http://wn.com/Lecture_6_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on the applications of least squares for the course, Introd...
- published: 09 Jul 2008
- views: 15999
- author: Stanford
Lecture 9 | Introduction to Linear Dynamical Systems
- Duration: 69:02
- Updated: 22 Jul 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems for the course, Intr...
http://wn.com/Lecture_9_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems for the course, Intr...
- published: 09 Jul 2008
- views: 14141
- author: Stanford
Lecture 10 | Introduction to Linear Dynamical Systems
- Duration: 71:42
- Updated: 16 Jul 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems and how they relate ...
http://wn.com/Lecture_10_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems and how they relate ...
- published: 09 Jul 2008
- views: 12782
- author: Stanford
Lecture 11 | Introduction to Linear Dynamical Systems
- Duration: 68:55
- Updated: 02 Jul 2013
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on how to find solutions via LaPlace transform and the use ...
http://wn.com/Lecture_11_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on how to find solutions via LaPlace transform and the use ...
- published: 09 Jul 2008
- views: 10336
- author: StanfordUniversity
Lecture 12 | Introduction to Linear Dynamical Systems
- Duration: 73:37
- Updated: 02 Jul 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on matrix exponentials, eigenvectors, and diagonalization a...
http://wn.com/Lecture_12_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on matrix exponentials, eigenvectors, and diagonalization a...
- published: 09 Jul 2008
- views: 12212
- author: Stanford
Lecture 13 | Introduction to Linear Dynamical Systems
- Duration: 73:01
- Updated: 24 Feb 2013
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on generalized eigenvectors, diagonalization, and Jordan ca...
http://wn.com/Lecture_13_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on generalized eigenvectors, diagonalization, and Jordan ca...
- published: 09 Jul 2008
- views: 11103
- author: StanfordUniversity
Lecture 15 | Introduction to Linear Dynamical Systems
- Duration: 69:01
- Updated: 04 Jul 2013
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on inputs and outputs of linear dynamical systems, as well ...
http://wn.com/Lecture_15_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on inputs and outputs of linear dynamical systems, as well ...
- published: 09 Jul 2008
- views: 7797
- author: StanfordUniversity
Homogeneous Systems of Linear Equations - Intro to Eigenvalue/Eigenvector Method
- Duration: 18:44
- Updated: 06 Nov 2013
Gives an overview of the notation and terminology used when working with linear systems of differential equations. Outlines the method to be used for finding eigenvalues and eigenvectors, which provide solutions for a system of equations.
http://wn.com/Homogeneous_Systems_of_Linear_Equations_-_Intro_to_Eigenvalue_Eigenvector_Method
Gives an overview of the notation and terminology used when working with linear systems of differential equations. Outlines the method to be used for finding eigenvalues and eigenvectors, which provide solutions for a system of equations.
- published: 06 Nov 2013
- views: 21
Differential Equations - Solve Linear System using Laplace transforms
- Duration: 14:48
- Updated: 23 Jul 2014
Solve the system: dx/dt + 3x + dy/dt = 1, dx/dt - x + dy/dt - y = e^t, x(0)=0, y(0)=0.
http://wn.com/Differential_Equations_-_Solve_Linear_System_using_Laplace_transforms
Solve the system: dx/dt + 3x + dy/dt = 1, dx/dt - x + dy/dt - y = e^t, x(0)=0, y(0)=0.
- published: 22 Jul 2013
- views: 1915
- author: ProfessorFife
Linearizing non-linear dynamic equations
- Duration: 7:27
- Updated: 23 Aug 2014
In this lecture, we go through the steps of linearizing non-linear differential equations about a given operating/equilibrium point. We will use the multi-va...
http://wn.com/Linearizing_non-linear_dynamic_equations
In this lecture, we go through the steps of linearizing non-linear differential equations about a given operating/equilibrium point. We will use the multi-va...
- published: 25 Sep 2012
- views: 8537
- author: rmjds
Partial differential equation
- Duration: 37:52
- Updated: 29 Aug 2014
In mathematics, a partial differential equation (PDE) is a differential equation that contains unknown multivariable functions and their partial derivatives....
http://wn.com/Partial_differential_equation
In mathematics, a partial differential equation (PDE) is a differential equation that contains unknown multivariable functions and their partial derivatives....
- published: 29 Aug 2014
- views: 2
- author: Audiopedia
Differential Equations, The Exponential Map Perspective - Lecture 7
- Duration: 40:08
- Updated: 11 Dec 2013
The seventh in a series of lectures which will examine differential equations from the perspective of the exponential map. The seventh lecture continues with...
http://wn.com/Differential_Equations,_The_Exponential_Map_Perspective_-_Lecture_7
The seventh in a series of lectures which will examine differential equations from the perspective of the exponential map. The seventh lecture continues with...
- published: 25 Oct 2013
- views: 40
- author: Brian Palmer
-
Lecture 1 | Introduction to Linear Dynamical Systems
Lecture 1 | Introduction to Linear Dynamical SystemsLecture 1 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives an overview of the course, Introduction to Linear Dynamical Sy... -
Differential Equations: The Exponential Map Perspective - Lecture 1
Differential Equations: The Exponential Map Perspective - Lecture 1Differential Equations: The Exponential Map Perspective - Lecture 1
The first in a series of lectures which will examine differential equations from the perspective of the exponential map. The first lecture starts by consider... -
Differential Equations: The Exponential Map Perspective - Lecture 6
Differential Equations: The Exponential Map Perspective - Lecture 6Differential Equations: The Exponential Map Perspective - Lecture 6
The sixth in a series of lectures which will examine differential equations from the perspective of the exponential map. The sixth lecture introduces very ba... -
Differential Equations, the Exponential Map Perspective - Lecture 11
Differential Equations, the Exponential Map Perspective - Lecture 11Differential Equations, the Exponential Map Perspective - Lecture 11
The eleventh in a series of lectures which will examine differential equations from the perspective of the exponential map. In the eleventh lecture we comput... -
Physics 111: Non-Linear Dynamics and Chaos (NLD)
Physics 111: Non-Linear Dynamics and Chaos (NLD)Physics 111: Non-Linear Dynamics and Chaos (NLD)
Physics 111 Advanced Laboratory This video accompanies the Non-Linear Dynamics and Chaos Experiment, providing students with an introduction to the theory, a... -
Introduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
Introduction to Nonlinear PDEs I. Nonlinear Diffusion EquationIntroduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
Professor Ugur Abdulla, Florida Institute of Technology View in HD on the FIT Site: http://media.fit.edu/load.php?fn=Math%20Seminar%20-%20Part%201-%20Dr.%20U...
Lecture 1 | Introduction to Linear Dynamical Systems
- Duration: 76:46
- Updated: 29 Aug 2014
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives an overview of the course, Introduction to Linear Dynamical Sy...
http://wn.com/Lecture_1_|_Introduction_to_Linear_Dynamical_Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives an overview of the course, Introduction to Linear Dynamical Sy...
- published: 09 Jul 2008
- views: 94697
- author: Stanford
Differential Equations: The Exponential Map Perspective - Lecture 1
- Duration: 48:25
- Updated: 18 Mar 2014
The first in a series of lectures which will examine differential equations from the perspective of the exponential map. The first lecture starts by consider...
http://wn.com/Differential_Equations_The_Exponential_Map_Perspective_-_Lecture_1
The first in a series of lectures which will examine differential equations from the perspective of the exponential map. The first lecture starts by consider...
- published: 06 Sep 2013
- views: 210
- author: Brian Palmer
Differential Equations: The Exponential Map Perspective - Lecture 6
- Duration: 38:20
- Updated: 29 Apr 2014
The sixth in a series of lectures which will examine differential equations from the perspective of the exponential map. The sixth lecture introduces very ba...
http://wn.com/Differential_Equations_The_Exponential_Map_Perspective_-_Lecture_6
The sixth in a series of lectures which will examine differential equations from the perspective of the exponential map. The sixth lecture introduces very ba...
- published: 18 Oct 2013
- views: 64
- author: Brian Palmer
Differential Equations, the Exponential Map Perspective - Lecture 11
- Duration: 41:31
- Updated: 17 Feb 2014
The eleventh in a series of lectures which will examine differential equations from the perspective of the exponential map. In the eleventh lecture we comput...
http://wn.com/Differential_Equations,_the_Exponential_Map_Perspective_-_Lecture_11
The eleventh in a series of lectures which will examine differential equations from the perspective of the exponential map. In the eleventh lecture we comput...
- published: 06 Dec 2013
- views: 55
- author: Brian Palmer
Physics 111: Non-Linear Dynamics and Chaos (NLD)
- Duration: 45:56
- Updated: 30 Aug 2014
Physics 111 Advanced Laboratory This video accompanies the Non-Linear Dynamics and Chaos Experiment, providing students with an introduction to the theory, a...
http://wn.com/Physics_111_Non-Linear_Dynamics_and_Chaos_(NLD)
Physics 111 Advanced Laboratory This video accompanies the Non-Linear Dynamics and Chaos Experiment, providing students with an introduction to the theory, a...
- published: 08 Mar 2012
- views: 5258
- author: UCBerkeley
Introduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
- Duration: 62:27
- Updated: 08 Aug 2014
Professor Ugur Abdulla, Florida Institute of Technology View in HD on the FIT Site: http://media.fit.edu/load.php?fn=Math%20Seminar%20-%20Part%201-%20Dr.%20U...
http://wn.com/Introduction_to_Nonlinear_PDEs_I._Nonlinear_Diffusion_Equation
Professor Ugur Abdulla, Florida Institute of Technology View in HD on the FIT Site: http://media.fit.edu/load.php?fn=Math%20Seminar%20-%20Part%201-%20Dr.%20U...
- published: 14 Nov 2013
- views: 2127
- author: FIT Mathematical Science
-
All About - Dynamical systems theory
All About - Dynamical systems theoryAll About - Dynamical systems theory
What is Dynamical systems theory? A report all about Dynamical systems theory for homework/assignment Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a cantor set—one gets dynamic equ -
Differential equation
Differential equationDifferential equation
A differential equation is a mathematical equation that relates some function of one or more variables with its derivatives. Differential equations arise whenever a deterministic relation involving some continuously varying quantities (modeled by functions) and their rates of change in space and/or time (expressed as derivatives) is known or postulated. Because such relations are extremely common, differential equations play a prominent role in many disciplines including engineering, physics, economics, and biology. Differential equations are mathematically studied from several different perspectives, mostly concerned with their solutions — t -
Interactive Exploration of a Dynamical System
Interactive Exploration of a Dynamical SystemInteractive Exploration of a Dynamical System
Interactive Exploration of a Dynamical System Follow us on Twitter - https://twitter.com/ExcellentVideos Facebook - http://www.facebook.com/excellent.randomv... -
Examining Dynamic Systems (Dr. Eric R. Kaufmann)
Examining Dynamic Systems (Dr. Eric R. Kaufmann)Examining Dynamic Systems (Dr. Eric R. Kaufmann)
Dr. Eric R. Kaufmann faces challenges everyday in his research even though he investigates ordinary differential equations. The associate professor leads the... -
Dynamical Modeling with PottersWheel
Dynamical Modeling with PottersWheelDynamical Modeling with PottersWheel
PottersWheel is a program to create differential equation based models of dynamical systems like biochemical reaction networks. It can fit a model to experim... -
Graphing Linear Equations2
Graphing Linear Equations2Graphing Linear Equations2
Graphing Linear Equations2 -
Non Linear Eiffel Tower Warp
Non Linear Eiffel Tower WarpNon Linear Eiffel Tower Warp
-
Lesson 6.5--Special Cases of Linear Systmes
Lesson 6.5--Special Cases of Linear SystmesLesson 6.5--Special Cases of Linear Systmes
-
Algebra 1: Fitting Equations to Data
Algebra 1: Fitting Equations to DataAlgebra 1: Fitting Equations to Data
Rim of the World Unified School District Math Teacher Mr. Dave Esquer Teaches Algebra 1 Math Studies! We hope these math videos help you out in your educational path, and can help you pass your tests! Comment below and help others with questions, as we may not see the comments in time. Enjoy! Rim High School Social Media If you want a copy of these videos, please contact us for permission to download, as these videos are under the sole possession of Rim of the World Unified School District, and permission given to Rim of the World High School for Hosting on YouTube. rhssocial@rimsd.k12.ca.us -
Linear Applications 1
Linear Applications 1Linear Applications 1
This is the first video for Linear Applications on http://www.ulearniversity.com. -
Linear Applications 2
Linear Applications 2Linear Applications 2
This is the second video for Linear Applications on http://www.ulearniversity.com -
Booklet-System Dynamisch (T-Systems)
Booklet-System Dynamisch (T-Systems)Booklet-System Dynamisch (T-Systems)
This video was brought to you by www.unigraphica.com. Please visit our website for further information and many other brilliant innovation around the graphic... -
Section 2.2-2.4-Solving Linear Equations
Section 2.2-2.4-Solving Linear EquationsSection 2.2-2.4-Solving Linear Equations
-
NOTES 05.7 Linearization Ex 6
NOTES 05.7 Linearization Ex 6NOTES 05.7 Linearization Ex 6
-
Linear Measurement- Capacity
Linear Measurement- CapacityLinear Measurement- Capacity
13
All About - Dynamical systems theory
- Duration: 2:09
- Updated: 10 Dec 2014
What is Dynamical systems theory?
A report all about Dynamical systems theory for homework/assignment
Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a cantor set—one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations.
Intro/Outro music:
Discovery Hit/Chucky the Construction Worker - Kevin MacLeod (incompetech.com)
Licensed under CC-BY-3.0
Text derived from:
http://en.wikipedia.org/wiki/Dynamical_systems_theory
Text to Speech powered by voice-rss.com
Images are Public Domain or CC-BY-3.0:
2048px-Lorenz_attractor_yb.svg.png from http://en.wikipedia.org/wiki/Dynamical_systems_theory
240px-Lorenz_attractor_yb.svg.png from http://simple.wikipedia.org/wiki/Dynamical_systems_theory
2000px-Lorenz_attractor_yb.svg.png from http://zh.wikipedia.org/wiki/%E5%8A%A8%E6%80%81%E7%B3%BB%E7%BB%9F%E7%90%86%E8%AE%BA
150px-Complex_systems_organizational_map.jpg from http://en.wikipedia.org/wiki/Complex_systems
http://wn.com/All_About_-_Dynamical_systems_theory
What is Dynamical systems theory?
A report all about Dynamical systems theory for homework/assignment
Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a cantor set—one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations.
Intro/Outro music:
Discovery Hit/Chucky the Construction Worker - Kevin MacLeod (incompetech.com)
Licensed under CC-BY-3.0
Text derived from:
http://en.wikipedia.org/wiki/Dynamical_systems_theory
Text to Speech powered by voice-rss.com
Images are Public Domain or CC-BY-3.0:
2048px-Lorenz_attractor_yb.svg.png from http://en.wikipedia.org/wiki/Dynamical_systems_theory
240px-Lorenz_attractor_yb.svg.png from http://simple.wikipedia.org/wiki/Dynamical_systems_theory
2000px-Lorenz_attractor_yb.svg.png from http://zh.wikipedia.org/wiki/%E5%8A%A8%E6%80%81%E7%B3%BB%E7%BB%9F%E7%90%86%E8%AE%BA
150px-Complex_systems_organizational_map.jpg from http://en.wikipedia.org/wiki/Complex_systems
- published: 10 Dec 2014
- views: 0
Differential equation
- Duration: 14:34
- Updated: 11 Aug 2014
A differential equation is a mathematical equation that relates some function of one or more variables with its derivatives. Differential equations arise whenever a deterministic relation involving some continuously varying quantities (modeled by functions) and their rates of change in space and/or time (expressed as derivatives) is known or postulated. Because such relations are extremely common, differential equations play a prominent role in many disciplines including engineering, physics, economics, and biology.
Differential equations are mathematically studied from several different perspectives, mostly concerned with their solutions — the set of functions that satisfy the equation. Only the simplest differential equations are solvable by explicit formulas; however, some properties of solutions of a given differential equation may be determined without finding their exact form. If a self-contained formula for the solution is not available, the solution may be numerically approximated using computers. The theory of dynamical systems puts emphasis on qualitative analysis of systems described by differential equations, while many numerical methods have been developed to determine solutions with a given degree of accuracy.
This video is targeted to blind users.
Attribution:
Article text available under CC-BY-SA
Creative Commons image source in video
http://wn.com/Differential_equation
A differential equation is a mathematical equation that relates some function of one or more variables with its derivatives. Differential equations arise whenever a deterministic relation involving some continuously varying quantities (modeled by functions) and their rates of change in space and/or time (expressed as derivatives) is known or postulated. Because such relations are extremely common, differential equations play a prominent role in many disciplines including engineering, physics, economics, and biology.
Differential equations are mathematically studied from several different perspectives, mostly concerned with their solutions — the set of functions that satisfy the equation. Only the simplest differential equations are solvable by explicit formulas; however, some properties of solutions of a given differential equation may be determined without finding their exact form. If a self-contained formula for the solution is not available, the solution may be numerically approximated using computers. The theory of dynamical systems puts emphasis on qualitative analysis of systems described by differential equations, while many numerical methods have been developed to determine solutions with a given degree of accuracy.
This video is targeted to blind users.
Attribution:
Article text available under CC-BY-SA
Creative Commons image source in video
- published: 11 Aug 2014
- views: 0
Interactive Exploration of a Dynamical System
- Duration: 5:47
- Updated: 07 Dec 2013
Interactive Exploration of a Dynamical System Follow us on Twitter - https://twitter.com/ExcellentVideos Facebook - http://www.facebook.com/excellent.randomv...
http://wn.com/Interactive_Exploration_of_a_Dynamical_System
Interactive Exploration of a Dynamical System Follow us on Twitter - https://twitter.com/ExcellentVideos Facebook - http://www.facebook.com/excellent.randomv...
- published: 06 Oct 2013
- views: 35
- author: ExcellentVideosOnly
Examining Dynamic Systems (Dr. Eric R. Kaufmann)
- Duration: 0:50
- Updated: 17 Aug 2014
Dr. Eric R. Kaufmann faces challenges everyday in his research even though he investigates ordinary differential equations. The associate professor leads the...
http://wn.com/Examining_Dynamic_Systems_(Dr._Eric_R._Kaufmann)
Dr. Eric R. Kaufmann faces challenges everyday in his research even though he investigates ordinary differential equations. The associate professor leads the...
- published: 04 Jun 2009
- views: 405
- author: UALRVIDEO
Dynamical Modeling with PottersWheel
- Duration: 7:53
- Updated: 26 Aug 2010
PottersWheel is a program to create differential equation based models of dynamical systems like biochemical reaction networks. It can fit a model to experim...
http://wn.com/Dynamical_Modeling_with_PottersWheel
PottersWheel is a program to create differential equation based models of dynamical systems like biochemical reaction networks. It can fit a model to experim...
- published: 02 Aug 2008
- views: 371
- author: tmaiwald
Graphing Linear Equations2
- Duration: 27:34
- Updated: 12 Sep 2013
Graphing Linear Equations2
http://wn.com/Graphing_Linear_Equations2
Graphing Linear Equations2
- published: 12 Sep 2013
- views: 3
Non Linear Eiffel Tower Warp
- Duration: 0:04
- Updated: 09 May 2012
http://wn.com/Non_Linear_Eiffel_Tower_Warp
- published: 09 May 2012
- views: 25
Lesson 6.5--Special Cases of Linear Systmes
- Duration: 14:24
- Updated: 25 Feb 2014
http://wn.com/Lesson_6.5--Special_Cases_of_Linear_Systmes
Algebra 1: Fitting Equations to Data
- Duration: 4:58
- Updated: 06 May 2014
Rim of the World Unified School District Math Teacher Mr. Dave Esquer Teaches Algebra 1 Math Studies!
We hope these math videos help you out in your educational path, and can help you pass your tests! Comment below and help others with questions, as we may not see the comments in time.
Enjoy!
Rim High School Social Media
If you want a copy of these videos, please contact us for permission to download, as these videos are under the sole possession of Rim of the World Unified School District, and permission given to Rim of the World High School for Hosting on YouTube.
rhssocial@rimsd.k12.ca.us
http://wn.com/Algebra_1_Fitting_Equations_to_Data
Rim of the World Unified School District Math Teacher Mr. Dave Esquer Teaches Algebra 1 Math Studies!
We hope these math videos help you out in your educational path, and can help you pass your tests! Comment below and help others with questions, as we may not see the comments in time.
Enjoy!
Rim High School Social Media
If you want a copy of these videos, please contact us for permission to download, as these videos are under the sole possession of Rim of the World Unified School District, and permission given to Rim of the World High School for Hosting on YouTube.
rhssocial@rimsd.k12.ca.us
- published: 06 May 2014
- views: 2
Linear Applications 1
- Duration: 3:46
- Updated: 22 Dec 2013
This is the first video for Linear Applications on http://www.ulearniversity.com.
http://wn.com/Linear_Applications_1
This is the first video for Linear Applications on http://www.ulearniversity.com.
- published: 22 Dec 2013
- views: 10
Linear Applications 2
- Duration: 4:13
- Updated: 22 Dec 2013
This is the second video for Linear Applications on http://www.ulearniversity.com
http://wn.com/Linear_Applications_2
This is the second video for Linear Applications on http://www.ulearniversity.com
- published: 22 Dec 2013
- views: 7
Booklet-System Dynamisch (T-Systems)
- Duration: 3:24
- Updated: 23 Feb 2011
This video was brought to you by www.unigraphica.com. Please visit our website for further information and many other brilliant innovation around the graphic...
http://wn.com/Booklet-System_Dynamisch_(T-Systems)
This video was brought to you by www.unigraphica.com. Please visit our website for further information and many other brilliant innovation around the graphic...
- published: 28 Oct 2010
- views: 75
- author: Unigraphica
Section 2.2-2.4-Solving Linear Equations
- Duration: 14:52
- Updated: 13 Oct 2014
http://wn.com/Section_2.2-2.4-Solving_Linear_Equations
- published: 13 Oct 2014
- views: 0
NOTES 05.7 Linearization Ex 6
- Duration: 4:49
- Updated: 05 Dec 2013
http://wn.com/NOTES_05.7_Linearization_Ex_6
- published: 05 Dec 2013
- views: 49
Linear Measurement- Capacity
- Duration: 0:43
- Updated: 16 Dec 2013
13
http://wn.com/Linear_Measurement-_Capacity
13
- published: 16 Dec 2013
- views: 3
- Playlist
- Chat
50:27
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Sketching Solutions of 2x2 Homogeneous Linear System with Constant Coefficients View the c...
published: 17 Jan 2008
author: MIT OpenCourseWare
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Lec 27 | MIT 18.03 Differential Equations, Spring 2006
Sketching Solutions of 2x2 Homogeneous Linear System with Constant Coefficients View the complete course: http://ocw.mit.edu/18-03S06 License: Creative Commo...- published: 17 Jan 2008
- views: 52637
- author: MIT OpenCourseWare
47:11
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the...
published: 17 Jan 2008
author: MIT OpenCourseWare
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Lec 31 | MIT 18.03 Differential Equations, Spring 2006
Non-linear Autonomous Systems: Finding the Critical Points and Sketching Trajectories; the Non-linear Pendulum. View the complete course: http://ocw.mit.edu/...- published: 17 Jan 2008
- views: 33293
- author: MIT OpenCourseWare
65:34
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
Discussion of differential equations as a representation of dynamic systems. Introduction ...
published: 18 Aug 2013
author: Rick Hill
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
System Dynamics and Control: Module 3 - Mathematical Modeling Part I
Discussion of differential equations as a representation of dynamic systems. Introduction to the Laplace Transform as a tool for solving differential equations.- published: 18 Aug 2013
- views: 4699
- author: Rick Hill
56:46
Multiagent Dynamical Systems
I will show how to model multiagent systems using dynamical systems theory by deriving a c...
published: 04 May 2012
author: Bill Broadley
Multiagent Dynamical Systems
Multiagent Dynamical Systems
I will show how to model multiagent systems using dynamical systems theory by deriving a class of macroscopic differential equations that describe mutual ada...- published: 04 May 2012
- views: 1356
- author: Bill Broadley
18:01
Fixed points and stability of a nonlinear system
Find the fixed points and determines the linear stability of a system of two first-order n...
published: 11 Nov 2013
author: Prof. Jeffrey Chasnov
Fixed points and stability of a nonlinear system
Fixed points and stability of a nonlinear system
Find the fixed points and determines the linear stability of a system of two first-order nonlinear differential equations. Lecture notes at http://www.math.u...- published: 11 Nov 2013
- views: 2789
- author: Prof. Jeffrey Chasnov
101:13
Dynamical Systems
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - cha...
published: 27 Oct 2012
author: Stanford Complexity Group
Dynamical Systems
Dynamical Systems
Mathematics of Complexity lecture 3 Class description: We've all heard the buzzwords - chaos, fractals, networks, power laws. What do these terms mean in a r...- published: 27 Oct 2012
- views: 3436
- author: Stanford Complexity Group
47:15
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim Vie...
published: 02 Sep 2011
author: MIT OpenCourseWare
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations | MIT RES.6.007 Signals and Systems
Lecture 6, Systems Represented by Differential Equations Instructor: Alan V. Oppenheim View the complete course: http://ocw.mit.edu/RES-6.007S11 License: Cre...- published: 02 Sep 2011
- views: 16138
- author: MIT OpenCourseWare
9:01
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation Dynamic Systems....
published: 03 Aug 2011
author: 4DSCIENCE
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation ( Dynamic Systems ) | Mechanical Engineering
State Space Representation Dynamic Systems.- published: 03 Aug 2011
- views: 28006
- author: 4DSCIENCE
53:31
Logical Analysis of Hybrid Systems
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Departme...
published: 17 Mar 2011
author: cmurobotics
Logical Analysis of Hybrid Systems
Logical Analysis of Hybrid Systems
RI Seminar, February 18, 2011 Andre Platzer Assistant Professor, Computer Science Department, Carnegie Mellon University Hybrid systems model cyber-physical ...- published: 17 Mar 2011
- views: 972
- author: cmurobotics
65:52
Lecture 2 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: Stanford
Lecture 2 | Introduction to Linear Dynamical Systems
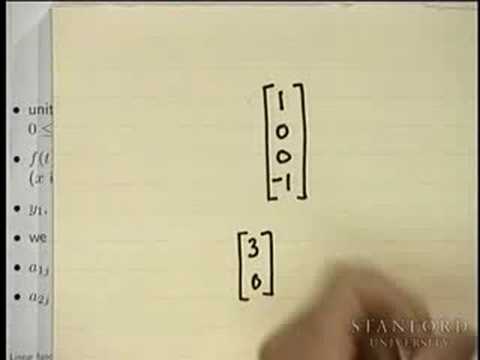
Lecture 2 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on linear functions for the course, Introduction to Linear ...- published: 09 Jul 2008
- views: 45047
- author: Stanford
79:11
Lecture 3 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, g...
published: 09 Jul 2008
author: Stanford
Lecture 3 | Introduction to Linear Dynamical Systems
Lecture 3 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives a review of linear algebra for the course, Introduction to Lin...- published: 09 Jul 2008
- views: 28340
- author: Stanford
76:19
Lecture 6 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: Stanford
Lecture 6 | Introduction to Linear Dynamical Systems
Lecture 6 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on the applications of least squares for the course, Introd...- published: 09 Jul 2008
- views: 15999
- author: Stanford
69:02
Lecture 9 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: Stanford
Lecture 9 | Introduction to Linear Dynamical Systems
Lecture 9 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems for the course, Intr...- published: 09 Jul 2008
- views: 14141
- author: Stanford
71:42
Lecture 10 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: Stanford
Lecture 10 | Introduction to Linear Dynamical Systems
Lecture 10 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on autonomous linear dynamical systems and how they relate ...- published: 09 Jul 2008
- views: 12782
- author: Stanford
Youtube results:
68:55
Lecture 11 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: StanfordUniversity
Lecture 11 | Introduction to Linear Dynamical Systems
Lecture 11 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on how to find solutions via LaPlace transform and the use ...- published: 09 Jul 2008
- views: 10336
- author: StanfordUniversity
73:37
Lecture 12 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: Stanford
Lecture 12 | Introduction to Linear Dynamical Systems
Lecture 12 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on matrix exponentials, eigenvectors, and diagonalization a...- published: 09 Jul 2008
- views: 12212
- author: Stanford
73:01
Lecture 13 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: StanfordUniversity
Lecture 13 | Introduction to Linear Dynamical Systems
Lecture 13 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on generalized eigenvectors, diagonalization, and Jordan ca...- published: 09 Jul 2008
- views: 11103
- author: StanfordUniversity
69:01
Lecture 15 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, l...
published: 09 Jul 2008
author: StanfordUniversity
Lecture 15 | Introduction to Linear Dynamical Systems
Lecture 15 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, lectures on inputs and outputs of linear dynamical systems, as well ...- published: 09 Jul 2008
- views: 7797
- author: StanfordUniversity
- Playlist
- Chat
76:46
Lecture 1 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, g...
published: 09 Jul 2008
author: Stanford
Lecture 1 | Introduction to Linear Dynamical Systems
Lecture 1 | Introduction to Linear Dynamical Systems
Professor Stephen Boyd, of the Electrical Engineering department at Stanford University, gives an overview of the course, Introduction to Linear Dynamical Sy...- published: 09 Jul 2008
- views: 94697
- author: Stanford
48:25
Differential Equations: The Exponential Map Perspective - Lecture 1
The first in a series of lectures which will examine differential equations from the persp...
published: 06 Sep 2013
author: Brian Palmer
Differential Equations: The Exponential Map Perspective - Lecture 1
Differential Equations: The Exponential Map Perspective - Lecture 1
The first in a series of lectures which will examine differential equations from the perspective of the exponential map. The first lecture starts by consider...- published: 06 Sep 2013
- views: 210
- author: Brian Palmer
38:20
Differential Equations: The Exponential Map Perspective - Lecture 6
The sixth in a series of lectures which will examine differential equations from the persp...
published: 18 Oct 2013
author: Brian Palmer
Differential Equations: The Exponential Map Perspective - Lecture 6
Differential Equations: The Exponential Map Perspective - Lecture 6
The sixth in a series of lectures which will examine differential equations from the perspective of the exponential map. The sixth lecture introduces very ba...- published: 18 Oct 2013
- views: 64
- author: Brian Palmer
41:31
Differential Equations, the Exponential Map Perspective - Lecture 11
The eleventh in a series of lectures which will examine differential equations from the pe...
published: 06 Dec 2013
author: Brian Palmer
Differential Equations, the Exponential Map Perspective - Lecture 11
Differential Equations, the Exponential Map Perspective - Lecture 11
The eleventh in a series of lectures which will examine differential equations from the perspective of the exponential map. In the eleventh lecture we comput...- published: 06 Dec 2013
- views: 55
- author: Brian Palmer
45:56
Physics 111: Non-Linear Dynamics and Chaos (NLD)
Physics 111 Advanced Laboratory This video accompanies the Non-Linear Dynamics and Chaos E...
published: 08 Mar 2012
author: UCBerkeley
Physics 111: Non-Linear Dynamics and Chaos (NLD)
Physics 111: Non-Linear Dynamics and Chaos (NLD)
Physics 111 Advanced Laboratory This video accompanies the Non-Linear Dynamics and Chaos Experiment, providing students with an introduction to the theory, a...- published: 08 Mar 2012
- views: 5258
- author: UCBerkeley
62:27
Introduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
Professor Ugur Abdulla, Florida Institute of Technology View in HD on the FIT Site: http:/...
published: 14 Nov 2013
author: FIT Mathematical Science
Introduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
Introduction to Nonlinear PDEs I. Nonlinear Diffusion Equation
Professor Ugur Abdulla, Florida Institute of Technology View in HD on the FIT Site: http://media.fit.edu/load.php?fn=Math%20Seminar%20-%20Part%201-%20Dr.%20U...- published: 14 Nov 2013
- views: 2127
- author: FIT Mathematical Science
- Playlist
- Chat
2:09
All About - Dynamical systems theory
What is Dynamical systems theory?
A report all about Dynamical systems theory for homewor...
published: 10 Dec 2014
All About - Dynamical systems theory
All About - Dynamical systems theory
What is Dynamical systems theory? A report all about Dynamical systems theory for homework/assignment Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a cantor set—one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations. Intro/Outro music: Discovery Hit/Chucky the Construction Worker - Kevin MacLeod (incompetech.com) Licensed under CC-BY-3.0 Text derived from: http://en.wikipedia.org/wiki/Dynamical_systems_theory Text to Speech powered by voice-rss.com Images are Public Domain or CC-BY-3.0: 2048px-Lorenz_attractor_yb.svg.png from http://en.wikipedia.org/wiki/Dynamical_systems_theory 240px-Lorenz_attractor_yb.svg.png from http://simple.wikipedia.org/wiki/Dynamical_systems_theory 2000px-Lorenz_attractor_yb.svg.png from http://zh.wikipedia.org/wiki/%E5%8A%A8%E6%80%81%E7%B3%BB%E7%BB%9F%E7%90%86%E8%AE%BA 150px-Complex_systems_organizational_map.jpg from http://en.wikipedia.org/wiki/Complex_systems- published: 10 Dec 2014
- views: 0
14:34
Differential equation
A differential equation is a mathematical equation that relates some function of one or mo...
published: 11 Aug 2014
Differential equation
Differential equation
A differential equation is a mathematical equation that relates some function of one or more variables with its derivatives. Differential equations arise whenever a deterministic relation involving some continuously varying quantities (modeled by functions) and their rates of change in space and/or time (expressed as derivatives) is known or postulated. Because such relations are extremely common, differential equations play a prominent role in many disciplines including engineering, physics, economics, and biology. Differential equations are mathematically studied from several different perspectives, mostly concerned with their solutions — the set of functions that satisfy the equation. Only the simplest differential equations are solvable by explicit formulas; however, some properties of solutions of a given differential equation may be determined without finding their exact form. If a self-contained formula for the solution is not available, the solution may be numerically approximated using computers. The theory of dynamical systems puts emphasis on qualitative analysis of systems described by differential equations, while many numerical methods have been developed to determine solutions with a given degree of accuracy. This video is targeted to blind users. Attribution: Article text available under CC-BY-SA Creative Commons image source in video- published: 11 Aug 2014
- views: 0
5:47
Interactive Exploration of a Dynamical System
Interactive Exploration of a Dynamical System Follow us on Twitter - https://twitter.com/E...
published: 06 Oct 2013
author: ExcellentVideosOnly
Interactive Exploration of a Dynamical System
Interactive Exploration of a Dynamical System
Interactive Exploration of a Dynamical System Follow us on Twitter - https://twitter.com/ExcellentVideos Facebook - http://www.facebook.com/excellent.randomv...- published: 06 Oct 2013
- views: 35
- author: ExcellentVideosOnly
0:50
Examining Dynamic Systems (Dr. Eric R. Kaufmann)
Dr. Eric R. Kaufmann faces challenges everyday in his research even though he investigates...
published: 04 Jun 2009
author: UALRVIDEO
Examining Dynamic Systems (Dr. Eric R. Kaufmann)
Examining Dynamic Systems (Dr. Eric R. Kaufmann)
Dr. Eric R. Kaufmann faces challenges everyday in his research even though he investigates ordinary differential equations. The associate professor leads the...- published: 04 Jun 2009
- views: 405
- author: UALRVIDEO
7:53
Dynamical Modeling with PottersWheel
PottersWheel is a program to create differential equation based models of dynamical system...
published: 02 Aug 2008
author: tmaiwald
Dynamical Modeling with PottersWheel
Dynamical Modeling with PottersWheel
PottersWheel is a program to create differential equation based models of dynamical systems like biochemical reaction networks. It can fit a model to experim...- published: 02 Aug 2008
- views: 371
- author: tmaiwald
14:24
Lesson 6.5--Special Cases of Linear Systmes
...
published: 24 Feb 2014
author: Michael McDowell
Lesson 6.5--Special Cases of Linear Systmes
Lesson 6.5--Special Cases of Linear Systmes
- published: 24 Feb 2014
- views: 67
- author: Michael McDowell
4:58
Algebra 1: Fitting Equations to Data
Rim of the World Unified School District Math Teacher Mr. Dave Esquer Teaches Algebra 1 Ma...
published: 06 May 2014
Algebra 1: Fitting Equations to Data
Algebra 1: Fitting Equations to Data
Rim of the World Unified School District Math Teacher Mr. Dave Esquer Teaches Algebra 1 Math Studies! We hope these math videos help you out in your educational path, and can help you pass your tests! Comment below and help others with questions, as we may not see the comments in time. Enjoy! Rim High School Social Media If you want a copy of these videos, please contact us for permission to download, as these videos are under the sole possession of Rim of the World Unified School District, and permission given to Rim of the World High School for Hosting on YouTube. rhssocial@rimsd.k12.ca.us- published: 06 May 2014
- views: 2
3:24
Booklet-System Dynamisch (T-Systems)
This video was brought to you by www.unigraphica.com. Please visit our website for further...
published: 28 Oct 2010
author: Unigraphica
Booklet-System Dynamisch (T-Systems)
Booklet-System Dynamisch (T-Systems)
This video was brought to you by www.unigraphica.com. Please visit our website for further information and many other brilliant innovation around the graphic...- published: 28 Oct 2010
- views: 75
- author: Unigraphica
Youtube results:
photo: AP / Vincent Yu
11 Dec 2014
Whether one likes it or not, the umbrella movement has given Hong Kong a new spirit. Unlike the old spirit, whose raison d'être is economic development founded on political and social stability, this new spirit is based on non-financial values. Chief Executive Leung Chun-ying is correct in describing Occupy as a social movement on an unprecedented scale ... This new spirit is daring, iconoclastic and out of the box. Possibilities abound....
photo: US DoD / Cherie A. Thurlby
11 Dec 2014
Former US President George W Bush was "fully informed" about CIA interrogation techniques condemned in a Senate report, his vice-president says. Speaking to Fox News, Dick Cheney said Mr Bush "knew everything he needed to know" about the programme, and the was "full of crap". has defended its use of methods such as waterboarding on terror suspects after the 9/11 attacks ... Please turn on JavaScript ... ....
photo: AP / Jossy Ola
11 Dec 2014
Islamic State and Boko Haram behind most deaths, suggesting jihadism is moving from terrorism to more conventional fighting forces that try to gain or hold territory, report finds. Isis fighters parade through Mosul in an Iraqi army vehicle. Photograph. AP ... Iraq suffered 1,770 deaths, but the deadliest attacks were carried out in Nigeria, where just 27 incidents took 786 lives ... Sign up for the Guardian Today ... Sign up for the daily email. ....
« back to news headlines
Edit
The Australian
12 Dec 2014
Tertiary educated people have the skills and knowledge businesses need to be competitive in an increasingly dynamic and competitive global economy ... But this has not been accompanied by a systematic rethink of how we should design our tertiary system to best deliver to the majority ... These features would deliver a tertiary sector that is dynamic and diverse, where providers focus on their strengths to differentiate and specialise....
Edit
Syracuse
12 Dec 2014
politics ... By Jonathan Bernstein ... We've seen this dynamic again and again since Republicans took over the House in 2011, and it's not likely to change with the next Congress, despite the larger Republican majority ... Radicals want to differentiate themselves from mainstream conservatives, and mainstream conservatives don't want to show any daylight between themselves and anyone who can credibly claim to be True Conservatives ... ....
Edit
noodls
12 Dec 2014
The prototype of a drive module for electric hydrogen fuel cell drive systems will be presented for the first time ... The new BMW 6 Series Convertible, the new BMW 6 Series Coupe and the new BMW 6 Series Gran Coupe meet the highest expectations of a sporting luxury car in terms of driving dynamics, comfort, advanced technology and imposing elegance....
Edit
BMW at the North American International Auto Show (NAIAS) 2015 in Detroit. (BMW - Bayerische Motoren Werke AG)
noodls12 Dec 2014
The prototype of a drive module for electric hydrogen fuel cell drive systems will be presented for the first time ... The new BMW 6 Series Convertible, the new BMW 6 Series Coupe and the new BMW 6 Series Gran Coupe meet the highest expectations of a sporting luxury car in terms of driving dynamics, comfort, advanced technology and imposing elegance....
Edit
>Vistronix Bolsters National Intelligence and Data Analytics Footprint with Acquisition of ExaTech Solutions Inc.
Seattle Post12 Dec 2014
Vistronix, a leading provider of intelligence and technology solutions to national security agencies in the federal space, is pleased to announce that the company has finalized its acquisition of ExaTech Solutions, Inc., a Herndon-based information technology company providing software, systems and network engineering support to various customers in the Intelligence Community....
Edit
noodls
12 Dec 2014
Piotr Matyba has a unique research profile and studies the dynamics of electrons and molecules at surfaces, using table-top laser-like x-ray sources ... If we understand and are able to control the electronic and molecular dynamics on surfaces, we can develop catalytic systems that promote specific chemical reactions and outperform today's catalysts....
Edit
noodls
12 Dec 2014
The study of nearly 600 postings in a range of online health communities (OHCs) led by Dr Reeva Lederman from the Computational Bioinformatics and Health Information Systems Research Group, found there are thousands of OHCs where people share their health experiences with other people who may have experienced the same condition ... the Department of Computing and Information Systems....
Edit
HOW TO ELECT OR CHANGE THE CURRENCY FOR RECEIVING INTERIM DIVIDEND (PDF 300KB) (Dynam Japan Holdings Co Ltd)
noodls12 Dec 2014
Dynam Japan Holdings Co Ltd) ... ダイナムジャパンホールディングス DYNAM JAPAN HOLDINGS Co., Ltd.* ... This announcement is made by ダイナムジャパンホールディングス DYNAM JAPAN HOLDINGS Co., Ltd.* (the "Company") ... Dynam Japan Holdings Co., Ltd ... hiroaki_akaru@dynam.co.jp ... koji_takeshita@dynam.co.jp ... ダイナムジャパンホールディングス (DYNAM JAPAN HOLDINGS Co., Ltd.*) ... This noodl was issued by Dynam Japan Holdings Co....
Edit
>Vistronix Boosts Cloud Computing, Critical IT Systems and Specialized Mission Solutions Offerings with Acquisition of ...
Seattle Post12 Dec 2014
Founded in 2004, ACG provides highly technical solutions in the areas of cloud computing analytics, critical systems infrastructure and specialized mission solutions ... Founded in 1990, Vistronix provides national security information systems, solutions, and services that protect our nation’s safety, security and economic well-being through ingenuity, technology innovation and mission expertise....
Edit
noodls
12 Dec 2014
(Source. NRL - National Rugby League Ltd) ... And believe it or not, he's not bad at doing that, either. ... But the addition of a more dynamic hooker in Michael Lichaa could create more space on the flanks, while the option of Brett Morris at fullback will quicken up their long shifts, equating to more space and faster service to a player who should get the ball quicker and often. distributed by ... (noodl. 26241227) ....
Edit
South China Morning Post
12 Dec 2014
He also cautioned that it is important for students to keep in mind Hong Kong's political structure under "one country two systems" - especially the "one country" part ... Our definitions of universal suffrage differ - whether one person one vote equates genuine universal suffrage is debatable," said Lai from Scholarism....
- 1
- 2
- 3
- 4
- 5
- Next page »
